This is an old revision of the document!
Kubic Harmonics (K)
Download a notebook that generates this page
The kubic harmonics (also known as cubic harmonics) are linear combinations of the spherical harmonics and irreducible representations of the cubic ($O_h$) point group. For $s$, $p$ and $d$ wave-functions the kubic harmonics are besides a different order the same as the tesseral harmonics. For higher angular momentum they are different.
The relation between the kubic harmonics and the tesseral harmonics is given by the matrix $T_{Z\,\mathrm{to}\,K}$, whereby the vector $\{Z_{l}^{(m=-l)},K_{l}^{(m=-l+1)},\dots,K_{l}^{(m=l)}\}$ is given as $T_{Z\,\mathrm{to}\,K} \cdot \{Y_{l}^{(m=-l)},Y_{l}^{(m=-l+1)},\dots,Y_{l}^{(m=l)}\}$. Explicit forms of $T_{Z\,\mathrm{to}\,K}$ for $l$ equal or smaller to 6 are:
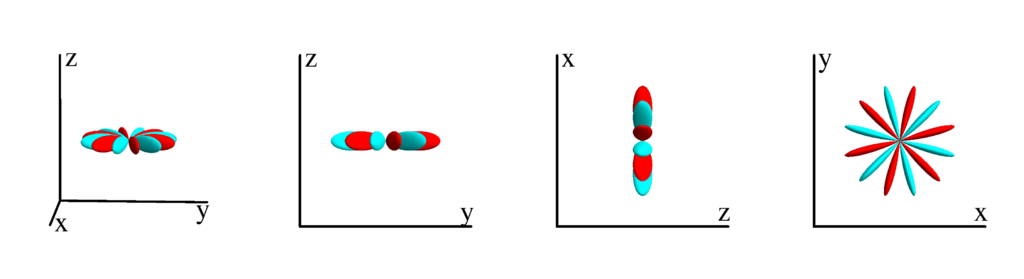
$$ \begin{align} &T_{Z\,\mathrm{to}\,K}^{l=0}=\left( \begin{array}{c} 1 \\ \end{array} \right) \\ \nonumber &T_{Z\,\mathrm{to}\,K}^{l=1}=\left( \begin{array}{ccc} 0 & 0 & 1 \\ 1 & 0 & 0 \\ 0 & 1 & 0 \\ \end{array} \right) \\ \nonumber &T_{Z\,\mathrm{to}\,K}^{l=2}=\left( \begin{array}{ccccc} 0 & 0 & 0 & 0 & 1 \\ 0 & 0 & 1 & 0 & 0 \\ 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 \\ 1 & 0 & 0 & 0 & 0 \\ \end{array} \right) \\ \nonumber &T_{Z\,\mathrm{to}\,K}^{l=3}=\left( \begin{array}{ccccccc} 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & -\frac{\sqrt{\frac{3}{2}}}{2} & 0 & \frac{\sqrt{\frac{5}{2}}}{2} \\ -\frac{\sqrt{\frac{5}{2}}}{2} & 0 & -\frac{\sqrt{\frac{3}{2}}}{2} & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & -\frac{\sqrt{\frac{5}{2}}}{2} & 0 & -\frac{\sqrt{\frac{3}{2}}}{2} \\ -\frac{\sqrt{\frac{3}{2}}}{2} & 0 & \frac{\sqrt{\frac{5}{2}}}{2} & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 & 0 \\ \end{array} \right) \\ \nonumber &T_{Z\,\mathrm{to}\,K}^{l=4}=\left( \begin{array}{ccccccccc} 0 & 0 & 0 & 0 & \frac{\sqrt{\frac{7}{3}}}{2} & 0 & 0 & 0 & \frac{\sqrt{\frac{5}{3}}}{2} \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & -\frac{\sqrt{\frac{5}{3}}}{2} & 0 & 0 & 0 & \frac{\sqrt{\frac{7}{3}}}{2} \\ 0 & -\frac{1}{2 \sqrt{2}} & 0 & -\frac{\sqrt{\frac{7}{2}}}{2} & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{\frac{7}{2}}}{2} & 0 & -\frac{1}{2 \sqrt{2}} & 0 \\ 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & \frac{\sqrt{\frac{7}{2}}}{2} & 0 & -\frac{1}{2 \sqrt{2}} & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & -\frac{1}{2 \sqrt{2}} & 0 & -\frac{\sqrt{\frac{7}{2}}}{2} & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ \end{array} \right) \\ \nonumber &T_{Z\,\mathrm{to}\,K}^{l=5}=\left( \begin{array}{ccccccccccc} 0 & 0 & 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{15}}{8} & 0 & -\frac{\sqrt{\frac{35}{2}}}{8} & 0 & \frac{3 \sqrt{\frac{7}{2}}}{8} \\ \frac{3 \sqrt{\frac{7}{2}}}{8} & 0 & \frac{\sqrt{\frac{35}{2}}}{8} & 0 & \frac{\sqrt{15}}{8} & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{21}}{8} & 0 & \frac{9}{8 \sqrt{2}} & 0 & \frac{\sqrt{\frac{5}{2}}}{8} \\ \frac{\sqrt{\frac{5}{2}}}{8} & 0 & -\frac{9}{8 \sqrt{2}} & 0 & \frac{\sqrt{21}}{8} & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{7}}{4} & 0 & -\frac{\sqrt{\frac{3}{2}}}{4} & 0 & -\frac{\sqrt{\frac{15}{2}}}{4} \\ \frac{\sqrt{\frac{15}{2}}}{4} & 0 & -\frac{\sqrt{\frac{3}{2}}}{4} & 0 & -\frac{\sqrt{7}}{4} & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ \end{array} \right) \\ \nonumber &T_{Z\,\mathrm{to}\,K}^{l=6}=\left( \begin{array}{ccccccccccccc} 0 & 0 & 0 & 0 & 0 & 0 & \frac{1}{2 \sqrt{2}} & 0 & 0 & 0 & -\frac{\sqrt{\frac{7}{2}}}{2} & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & -\frac{\sqrt{11}}{4} & 0 & 0 & 0 & \frac{\sqrt{5}}{4} \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{5}}{4} & 0 & 0 & 0 & \frac{\sqrt{11}}{4} \\ 0 & 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{\frac{7}{2}}}{2} & 0 & 0 & 0 & \frac{1}{2 \sqrt{2}} & 0 & 0 \\ 0 & -\frac{\sqrt{\frac{11}{2}}}{4} & 0 & -\frac{\sqrt{\frac{15}{2}}}{4} & 0 & \frac{\sqrt{3}}{4} & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & -\frac{\sqrt{3}}{4} & 0 & -\frac{\sqrt{\frac{15}{2}}}{4} & 0 & \frac{\sqrt{\frac{11}{2}}}{4} & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & \frac{\sqrt{165}}{16} & 0 & -\frac{9}{16} & 0 & \frac{\sqrt{\frac{5}{2}}}{8} & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & \frac{\sqrt{\frac{5}{2}}}{8} & 0 & \frac{9}{16} & 0 & \frac{\sqrt{165}}{16} & 0 \\ 0 & 0 & 0 & 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & \frac{\sqrt{3}}{16} & 0 & \frac{\sqrt{55}}{16} & 0 & \frac{3 \sqrt{\frac{11}{2}}}{8} & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & \frac{3 \sqrt{\frac{11}{2}}}{8} & 0 & -\frac{\sqrt{55}}{16} & 0 & \frac{\sqrt{3}}{16} & 0 \\ 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ \end{array} \right) \end{align} $$ ### The following table shows the kubic harmonics up to $l=6$. We list the explicit function in terms of the directional cosines $x$, $y$ and $z$. The plots show the surface defined by the equation $r={K_l^{(m)}}^* K_l^{(m)}$. The color of the surface is according to the phase with red for positive and cyan for negative. We show a 3D image as well as a projection along the $x$, $y$ and $z$ direction. ###
$l=0$
$m_l=0$ - $a_{1g}$
$$ K_{0}^{(0)}=\frac{1}{2 \sqrt{\pi }}\\ \phantom{K_{0}^{(0)}}=\frac{1}{2 \sqrt{\pi }} $$
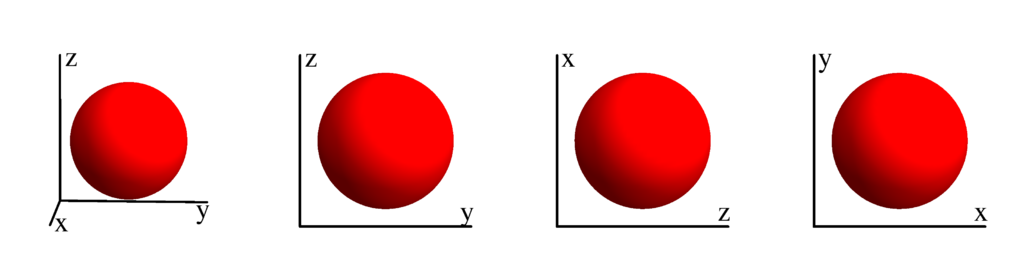
$l=1$
$m_l=-1$ - $t_{1u}$
$$ K_{1}^{(-1)}=\frac{1}{2} \sqrt{\frac{3}{\pi }} \sin (\theta ) \cos (\phi )\\ \phantom{K_{1}^{(-1)}}=\frac{1}{2} \sqrt{\frac{3}{\pi }} x $$
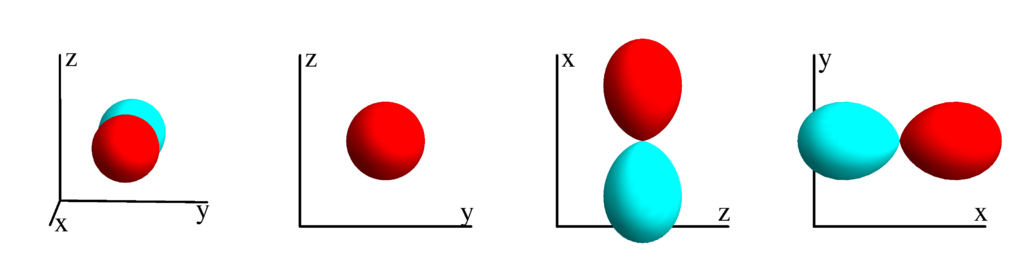
$m_l=0$ - $t_{1u}$
$$ K_{1}^{(0)}=\frac{1}{2} \sqrt{\frac{3}{\pi }} \sin (\theta ) \sin (\phi )\\ \phantom{K_{1}^{(0)}}=\frac{1}{2} \sqrt{\frac{3}{\pi }} y $$
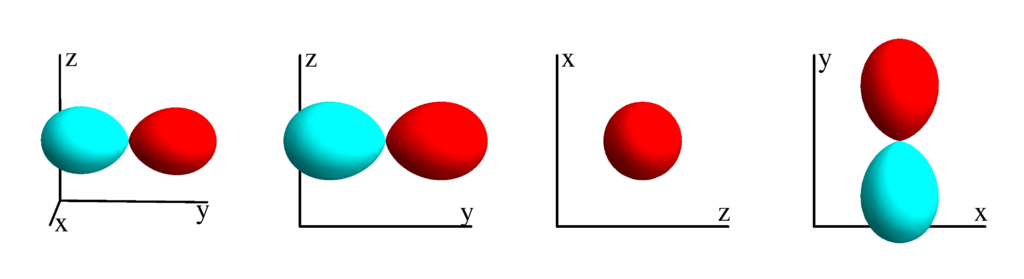
$m_l=1$ - $t_{1u}$
$$ K_{1}^{(1)}=\frac{1}{2} \sqrt{\frac{3}{\pi }} \cos (\theta )\\ \phantom{K_{1}^{(1)}}=\frac{1}{2} \sqrt{\frac{3}{\pi }} z $$

$l=2$
$m_l=-2$ - $e_g$
$$ K_{2}^{(-2)}=\frac{1}{4} \sqrt{\frac{15}{\pi }} \sin ^2(\theta ) \cos (2 \phi )\\ \phantom{K_{2}^{(-2)}}=\frac{1}{4} \sqrt{\frac{15}{\pi }} (x-y) (x+y) $$
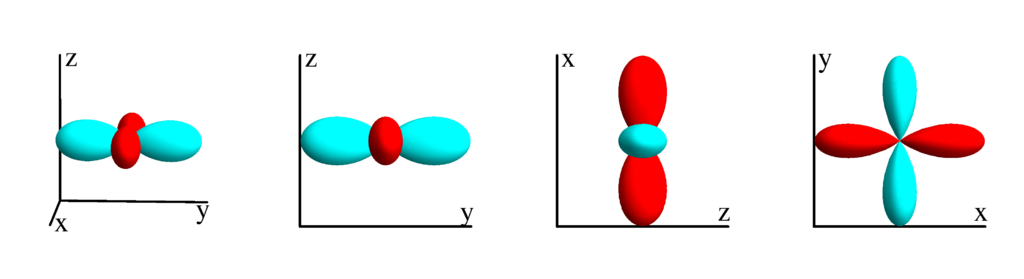
$m_l=-1$ - $e_g$
$$ K_{2}^{(-1)}=\frac{1}{8} \sqrt{\frac{5}{\pi }} (3 \cos (2 \theta )+1)\\ \phantom{K_{2}^{(-1)}}=-\frac{1}{4} \sqrt{\frac{5}{\pi }} \left(x^2+y^2-2 z^2\right) $$

$m_l=0$ - $t_{2g}$
$$ K_{2}^{(0)}=\frac{1}{2} \sqrt{\frac{15}{\pi }} \sin (\theta ) \cos (\theta ) \sin (\phi )\\ \phantom{K_{2}^{(0)}}=\frac{1}{2} \sqrt{\frac{15}{\pi }} y z $$
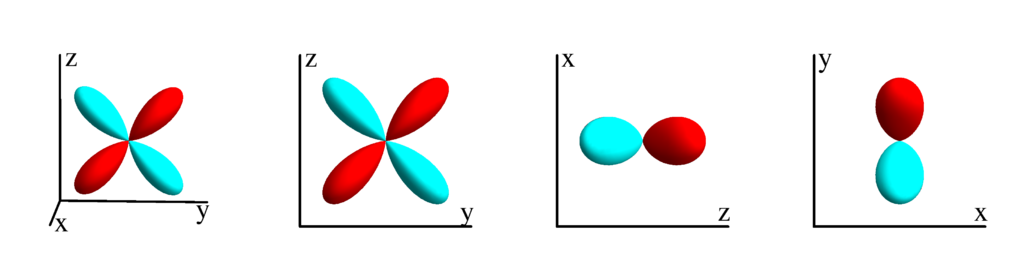
$m_l=1$ - $t_{2g}$
$$ K_{2}^{(1)}=\frac{1}{2} \sqrt{\frac{15}{\pi }} \sin (\theta ) \cos (\theta ) \cos (\phi )\\ \phantom{K_{2}^{(1)}}=\frac{1}{2} \sqrt{\frac{15}{\pi }} x z $$
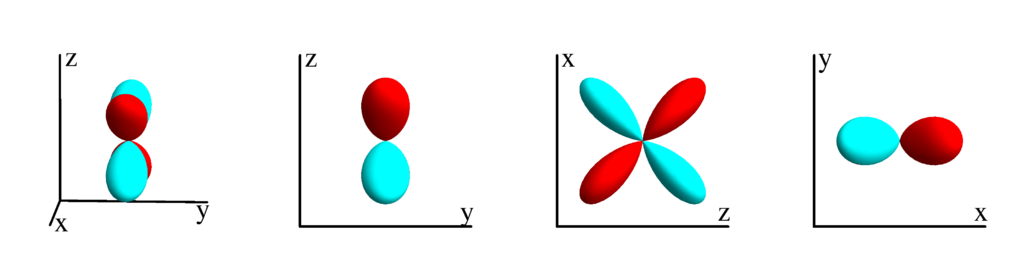
$m_l=2$ - $t_{2g}$
$$ K_{2}^{(2)}=\frac{1}{4} \sqrt{\frac{15}{\pi }} \sin ^2(\theta ) \sin (2 \phi )\\ \phantom{K_{2}^{(2)}}=\frac{1}{2} \sqrt{\frac{15}{\pi }} x y $$

$l=3$
$m_l=-3$ - $a_{2u}$
$$ K_{3}^{(-3)}=\frac{1}{4} \sqrt{\frac{105}{\pi }} \sin ^2(\theta ) \cos (\theta ) \sin (2 \phi )\\ \phantom{K_{3}^{(-3)}}=\frac{1}{2} \sqrt{\frac{105}{\pi }} x y z $$
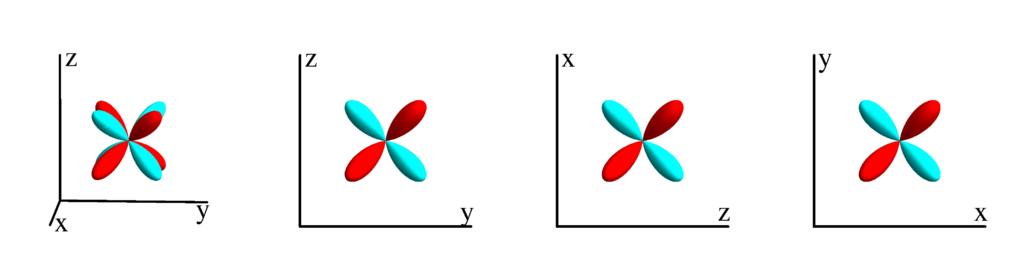
$m_l=-2$ - $t_{1u}$
$$ K_{3}^{(-2)}=-\frac{1}{64} \sqrt{\frac{7}{\pi }} \left(20 \sin (3 \theta ) \cos ^3(\phi )+3 \sin (\theta ) (\cos (\phi )-5 \cos (3 \phi ))\right)\\ \phantom{K_{3}^{(-2)}}=\frac{1}{4} \sqrt{\frac{7}{\pi }} x \left(2 x^2-3 \left(y^2+z^2\right)\right) $$
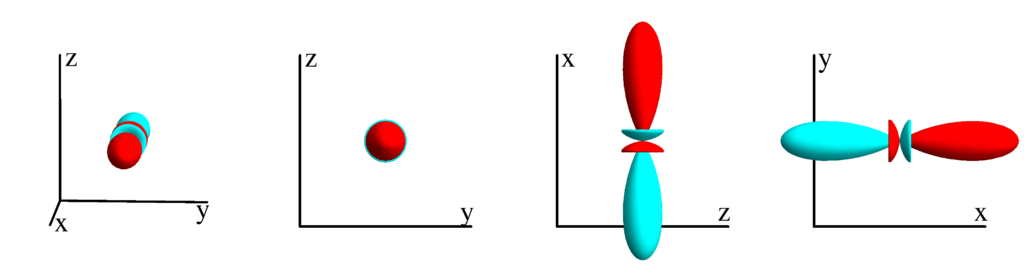
$m_l=-1$ - $t_{1u}$
$$ K_{3}^{(-1)}=-\frac{1}{64} \sqrt{\frac{7}{\pi }} \left(20 \sin ^3(\theta ) \sin (3 \phi )+3 (\sin (\theta )+5 \sin (3 \theta )) \sin (\phi )\right)\\ \phantom{K_{3}^{(-1)}}=\frac{1}{4} \sqrt{\frac{7}{\pi }} y \left(-3 x^2+2 y^2-3 z^2\right) $$

$m_l=0$ - $t_{1u}$
$$ K_{3}^{(0)}=\frac{1}{8} \sqrt{\frac{7}{\pi }} \cos (\theta ) (5 \cos (2 \theta )-1)\\ \phantom{K_{3}^{(0)}}=\frac{1}{4} \sqrt{\frac{7}{\pi }} z \left(2 z^2-3 \left(x^2+y^2\right)\right) $$

$m_l=1$ - $t_{2u}$
$$ K_{3}^{(1)}=-\frac{1}{16} \sqrt{\frac{105}{\pi }} \sin (\theta ) \cos (\phi ) \left(2 \sin ^2(\theta ) \cos (2 \phi )+3 \cos (2 \theta )+1\right)\\ \phantom{K_{3}^{(1)}}=\frac{1}{4} \sqrt{\frac{105}{\pi }} x (y-z) (y+z) $$

$m_l=2$ - $t_{2u}$
$$ K_{3}^{(2)}=\frac{1}{32} \sqrt{\frac{105}{\pi }} \sin (\theta ) \sin (\phi ) \left(-4 \sin ^2(\theta ) \cos (2 \phi )+6 \cos (2 \theta )+2\right)\\ \phantom{K_{3}^{(2)}}=\frac{1}{4} \sqrt{\frac{105}{\pi }} y \left(z^2-x^2\right) $$
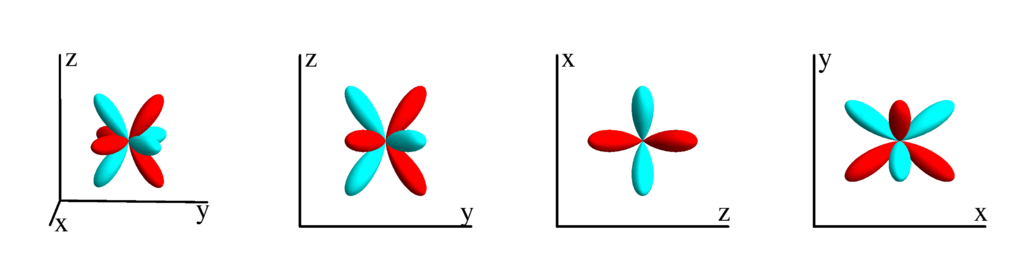
$m_l=3$ - $t_{2u}$
$$ K_{3}^{(3)}=\frac{1}{4} \sqrt{\frac{105}{\pi }} \sin ^2(\theta ) \cos (\theta ) \cos (2 \phi )\\ \phantom{K_{3}^{(3)}}=\frac{1}{4} \sqrt{\frac{105}{\pi }} z (x-y) (x+y) $$
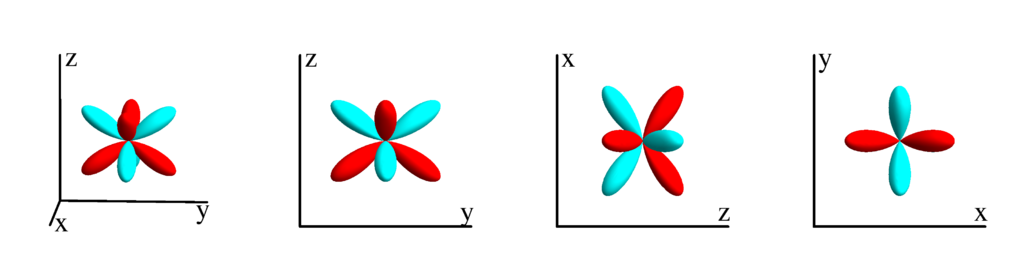
$l=4$
$m_l=-4$ - $a_{1g}$
$$ K_{4}^{(-4)}=\frac{1}{32} \sqrt{\frac{21}{\pi }} \left(5 \sin ^4(\theta ) \cos (4 \phi )+35 \cos ^4(\theta )-30 \cos ^2(\theta )+3\right)\\ \phantom{K_{4}^{(-4)}}=\frac{1}{4} \sqrt{\frac{21}{\pi }} \left(x^4-3 x^2 \left(y^2+z^2\right)+y^4-3 y^2 z^2+z^4\right) $$
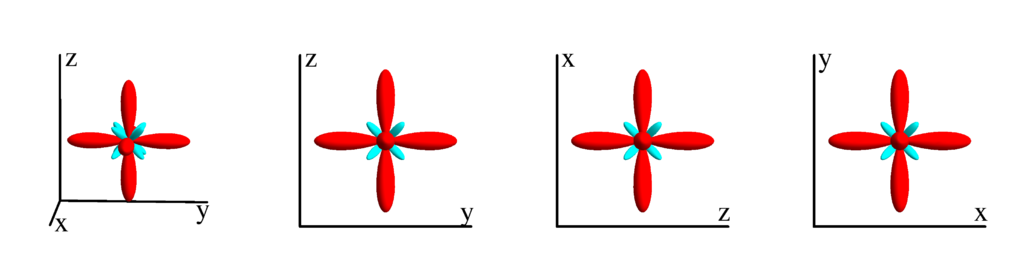
$m_l=-3$ - $e_g$
$$ K_{4}^{(-3)}=\frac{3}{16} \sqrt{\frac{5}{\pi }} \sin ^2(\theta ) (7 \cos (2 \theta )+5) \cos (2 \phi )\\ \phantom{K_{4}^{(-3)}}=-\frac{3}{8} \sqrt{\frac{5}{\pi }} (x-y) (x+y) \left(x^2+y^2-6 z^2\right) $$
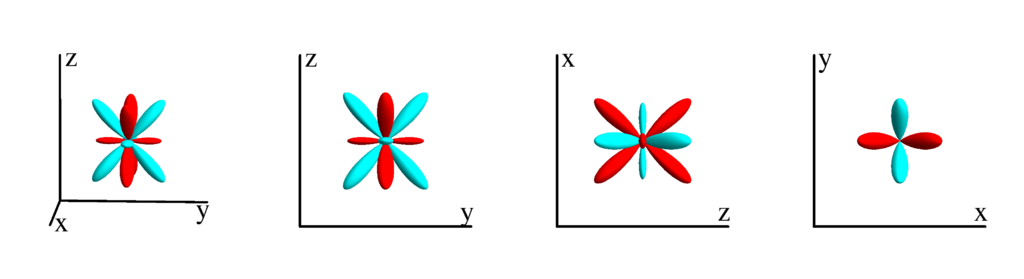
$m_l=-2$ - $e_g$
$$ K_{4}^{(-2)}=\frac{1}{32} \sqrt{\frac{15}{\pi }} \left(7 \sin ^4(\theta ) \cos (4 \phi )-35 \cos ^4(\theta )+30 \cos ^2(\theta )-3\right)\\ \phantom{K_{4}^{(-2)}}=\frac{1}{8} \sqrt{\frac{15}{\pi }} \left(x^4+6 z^2 \left(x^2+y^2\right)-12 x^2 y^2+y^4-2 z^4\right) $$

$m_l=-1$ - $t_{1g}$
$$ K_{4}^{(-1)}=-\frac{3}{32} \sqrt{\frac{35}{\pi }} \sin (2 \theta ) \sin (\phi ) \left(2 \sin ^2(\theta ) \cos (2 \phi )+3 \cos (2 \theta )+1\right)\\ \phantom{K_{4}^{(-1)}}=\frac{3}{4} \sqrt{\frac{35}{\pi }} y z (y-z) (y+z) $$
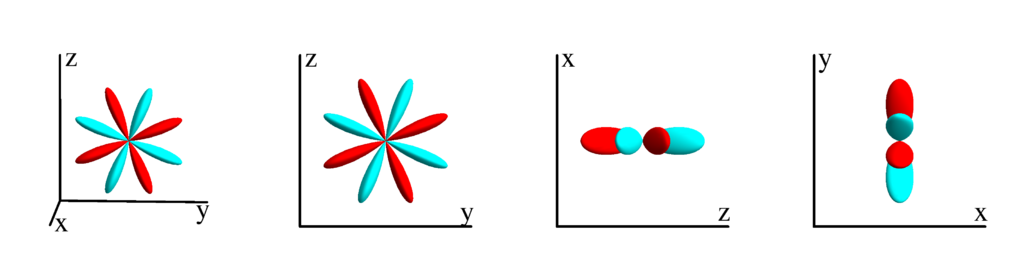
$m_l=0$ - $t_{1g}$
$$ K_{4}^{(0)}=\frac{3}{32} \sqrt{\frac{35}{\pi }} \sin (2 \theta ) \cos (\phi ) \left(-2 \sin ^2(\theta ) \cos (2 \phi )+3 \cos (2 \theta )+1\right)\\ \phantom{K_{4}^{(0)}}=\frac{3}{4} \sqrt{\frac{35}{\pi }} x z \left(z^2-x^2\right) $$
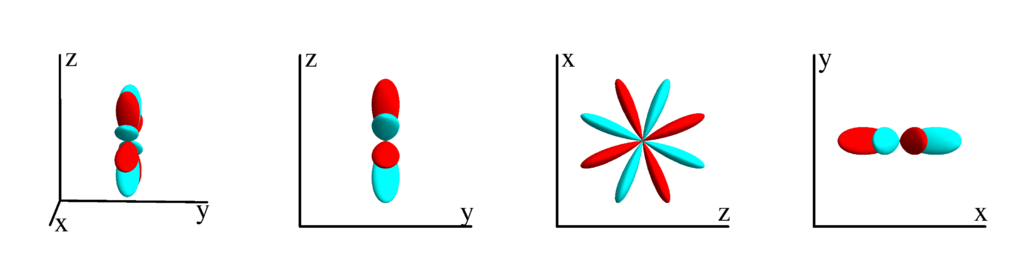
$m_l=1$ - $t_{1g}$
$$ K_{4}^{(1)}=\frac{3}{16} \sqrt{\frac{35}{\pi }} \sin ^4(\theta ) \sin (4 \phi )\\ \phantom{K_{4}^{(1)}}=\frac{3}{4} \sqrt{\frac{35}{\pi }} x y (x-y) (x+y) $$

$m_l=2$ - $t_{2g}$
$$ K_{4}^{(2)}=-\frac{3}{32} \sqrt{\frac{5}{\pi }} \sin (2 \theta ) \sin (\phi ) \left(-14 \sin ^2(\theta ) \cos (2 \phi )+7 \cos (2 \theta )-3\right)\\ \phantom{K_{4}^{(2)}}=-\frac{3}{4} \sqrt{\frac{5}{\pi }} y z \left(-6 x^2+y^2+z^2\right) $$

$m_l=3$ - $t_{2g}$
$$ K_{4}^{(3)}=-\frac{3}{64} \sqrt{\frac{5}{\pi }} (7 \sin (4 \theta ) \sin (\phi ) \sin (2 \phi )+\sin (2 \theta ) (\cos (\phi )+7 \cos (3 \phi )))\\ \phantom{K_{4}^{(3)}}=-\frac{3}{4} \sqrt{\frac{5}{\pi }} x z \left(x^2-6 y^2+z^2\right) $$
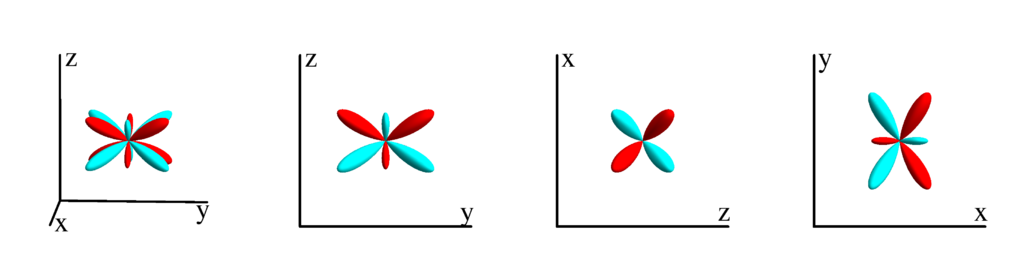
$m_l=4$ - $t_{2g}$
$$ K_{4}^{(4)}=\frac{3}{16} \sqrt{\frac{5}{\pi }} \sin ^2(\theta ) (7 \cos (2 \theta )+5) \sin (2 \phi )\\ \phantom{K_{4}^{(4)}}=-\frac{3}{4} \sqrt{\frac{5}{\pi }} x y \left(x^2+y^2-6 z^2\right) $$

$l=5$
$m_l=-5$ - $e_u$
$$ K_{5}^{(-5)}=\frac{1}{16} \sqrt{\frac{1155}{\pi }} \sin ^2(\theta ) \cos (\theta ) (3 \cos (2 \theta )+1) \sin (2 \phi )\\ \phantom{K_{5}^{(-5)}}=-\frac{1}{4} \sqrt{\frac{1155}{\pi }} x y z \left(x^2+y^2-2 z^2\right) $$

$m_l=-4$ - $e_u$
$$ K_{5}^{(-4)}=\frac{3}{16} \sqrt{\frac{385}{\pi }} \sin ^4(\theta ) \cos (\theta ) \sin (4 \phi )\\ \phantom{K_{5}^{(-4)}}=\frac{3}{4} \sqrt{\frac{385}{\pi }} x y z (x-y) (x+y) $$
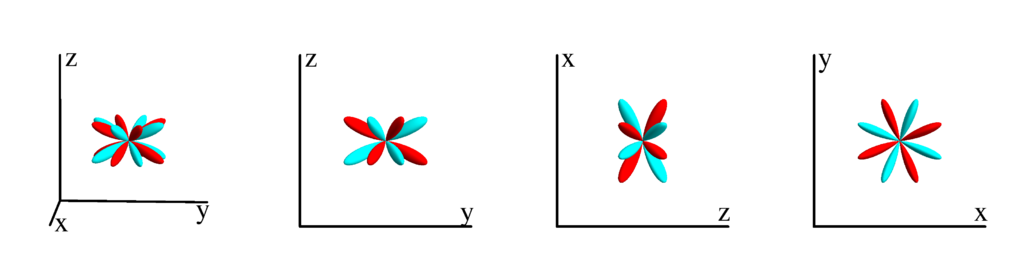
$m_l=-3$ - $t_{1u}^{(1)}$
$$ K_{5}^{(-3)}=\frac{1}{256} \sqrt{\frac{11}{\pi }} \sin (\theta ) \left(30 \left(21 \cos ^4(\theta )-14 \cos ^2(\theta )+1\right) \cos (\phi )+35 \sin ^2(\theta ) \left(1-9 \cos ^2(\theta )\right) \cos (3 \phi )+63 \sin ^4(\theta ) \cos (5 \phi )\right)\\ \phantom{K_{5}^{(-3)}}=\frac{1}{16} \sqrt{\frac{11}{\pi }} x \left(8 x^4-40 x^2 \left(y^2+z^2\right)+15 \left(y^2+z^2\right)^2\right) $$

$m_l=-2$ - $t_{1u}^{(1)}$
$$ K_{5}^{(-2)}=\frac{1}{256} \sqrt{\frac{11}{\pi }} \sin (\theta ) \left(63 \sin ^4(\theta ) \sin (5 \phi )+35 \sin ^2(\theta ) \left(9 \cos ^2(\theta )-1\right) \sin (3 \phi )+30 \left(21 \cos ^4(\theta )-14 \cos ^2(\theta )+1\right) \sin (\phi )\right)\\ \phantom{K_{5}^{(-2)}}=\frac{1}{16} \sqrt{\frac{11}{\pi }} y \left(15 x^4+x^2 \left(30 z^2-40 y^2\right)+8 y^4-40 y^2 z^2+15 z^4\right) $$

$m_l=-1$ - $t_{1u}^{(1)}$
$$ K_{5}^{(-1)}=\frac{1}{256} \sqrt{\frac{11}{\pi }} (30 \cos (\theta )+35 \cos (3 \theta )+63 \cos (5 \theta ))\\ \phantom{K_{5}^{(-1)}}=\frac{1}{16} \sqrt{\frac{11}{\pi }} z \left(-40 z^2 \left(x^2+y^2\right)+15 \left(x^2+y^2\right)^2+8 z^4\right) $$
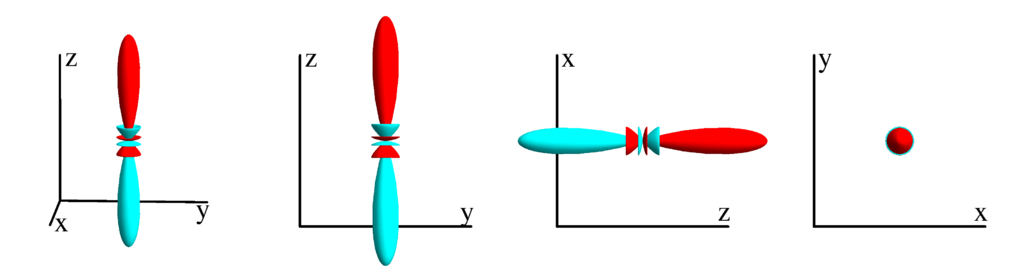
$m_l=0$ - $t_{1u}^{(2)}$
$$ K_{5}^{(0)}=\frac{3 \sqrt{\frac{385}{\pi }} \left(16 \sin (\theta ) (5 \cos (2 \phi )-3) \cos ^3(\phi )+\sin (3 \theta ) (14 \cos (\phi )+39 \cos (3 \phi )-5 \cos (5 \phi ))+\sin (5 \theta ) (42 \cos (\phi )-27 \cos (3 \phi )+\cos (5 \phi ))\right)}{4096}\\ \phantom{K_{5}^{(0)}}=\frac{3}{16} \sqrt{\frac{385}{\pi }} x \left(y^4-6 y^2 z^2+z^4\right) $$

$m_l=1$ - $t_{1u}^{(2)}$
$$ K_{5}^{(1)}=\frac{3 \sqrt{\frac{385}{\pi }} \left(8 \sin ^5(\theta ) \sin (5 \phi )+2 \sin (\theta ) \sin (\phi )+7 (\sin (3 \theta )+3 \sin (5 \theta )) \sin (\phi )-12 \sin ^3(\theta ) (9 \cos (2 \theta )+7) \sin (3 \phi )\right)}{2048}\\ \phantom{K_{5}^{(1)}}=\frac{3}{16} \sqrt{\frac{385}{\pi }} y \left(x^4-6 x^2 z^2+z^4\right) $$

$m_l=2$ - $t_{1u}^{(2)}$
$$ K_{5}^{(2)}=\frac{3}{16} \sqrt{\frac{385}{\pi }} \sin ^4(\theta ) \cos (\theta ) \cos (4 \phi )\\ \phantom{K_{5}^{(2)}}=\frac{3}{16} \sqrt{\frac{385}{\pi }} z \left(x^4-6 x^2 y^2+y^4\right) $$

$m_l=3$ - $t_{2u}$
$$ K_{5}^{(3)}=\frac{1}{128} \sqrt{\frac{1155}{\pi }} \sin (\theta ) \left(2 \left(21 \cos ^4(\theta )-14 \cos ^2(\theta )+1\right) \cos (\phi )+\sin ^2(\theta ) \left(1-9 \cos ^2(\theta )\right) \cos (3 \phi )-3 \sin ^4(\theta ) \cos (5 \phi )\right)\\ \phantom{K_{5}^{(3)}}=\frac{1}{8} \sqrt{\frac{1155}{\pi }} x \left(2 x^2 (y-z) (y+z)-y^4+z^4\right) $$

$m_l=4$ - $t_{2u}$
$$ K_{5}^{(4)}=\frac{1}{128} \sqrt{\frac{1155}{\pi }} \sin (\theta ) \left(\sin ^2(\theta ) \left(3 \sin ^2(\theta ) \sin (5 \phi )+\left(1-9 \cos ^2(\theta )\right) \sin (3 \phi )\right)+\left(-42 \cos ^4(\theta )+28 \cos ^2(\theta )-2\right) \sin (\phi )\right)\\ \phantom{K_{5}^{(4)}}=\frac{1}{8} \sqrt{\frac{1155}{\pi }} y (x-z) (x+z) \left(x^2-2 y^2+z^2\right) $$

$m_l=5$ - $t_{2u}$
$$ K_{5}^{(5)}=\frac{1}{16} \sqrt{\frac{1155}{\pi }} \sin ^2(\theta ) \cos (\theta ) (3 \cos (2 \theta )+1) \cos (2 \phi )\\ \phantom{K_{5}^{(5)}}=-\frac{1}{8} \sqrt{\frac{1155}{\pi }} z (x-y) (x+y) \left(x^2+y^2-2 z^2\right) $$

$l=6$
$m_l=-6$ - $a_{1g}$
$$ K_{6}^{(-6)}=\frac{1}{64} \sqrt{\frac{13}{2 \pi }} \left(21 \sin ^4(\theta ) \left(1-11 \cos ^2(\theta )\right) \cos (4 \phi )+231 \cos ^6(\theta )-315 \cos ^4(\theta )+105 \cos ^2(\theta )-5\right)\\ \phantom{K_{6}^{(-6)}}=\frac{1}{8} \sqrt{\frac{13}{2 \pi }} \left(2 x^6-15 x^4 \left(y^2+z^2\right)-15 x^2 \left(y^4-12 y^2 z^2+z^4\right)+\left(y^2+z^2\right) \left(2 y^4-17 y^2 z^2+2 z^4\right)\right) $$

$m_l=-5$ - $a_{2g}$
$$ K_{6}^{(-5)}=\frac{1}{128} \sqrt{\frac{15015}{2 \pi }} \sin ^2(\theta ) \left(\left(-33 \cos ^4(\theta )+18 \cos ^2(\theta )-1\right) \cos (2 \phi )+\sin ^4(\theta ) \cos (6 \phi )\right)\\ \phantom{K_{6}^{(-5)}}=-\frac{1}{8} \sqrt{\frac{15015}{2 \pi }} (x-y) (x+y) (x-z) (x+z) (y-z) (y+z) $$

$m_l=-4$ - $e_g$
$$ K_{6}^{(-4)}=\frac{1}{128} \sqrt{\frac{273}{2 \pi }} \sin ^2(\theta ) \left(5 \left(33 \cos ^4(\theta )-18 \cos ^2(\theta )+1\right) \cos (2 \phi )+11 \sin ^4(\theta ) \cos (6 \phi )\right)\\ \phantom{K_{6}^{(-4)}}=\frac{1}{8} \sqrt{\frac{273}{2 \pi }} (x-y) (x+y) \left(x^4-5 z^2 \left(x^2+y^2\right)-9 x^2 y^2+y^4+5 z^4\right) $$
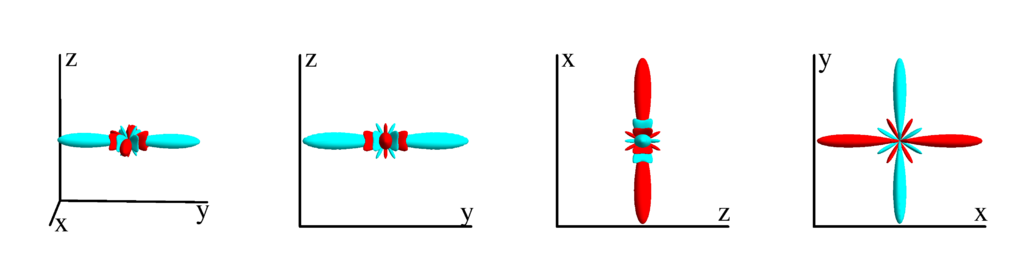
$m_l=-3$ - $e_g$
$$ K_{6}^{(-3)}=\frac{\sqrt{\frac{91}{2 \pi }} \left(48 \sin ^4(\theta ) (11 \cos (2 \theta )+9) \cos (4 \phi )+105 \cos (2 \theta )+126 \cos (4 \theta )+231 \cos (6 \theta )+50\right)}{2048}\\ \phantom{K_{6}^{(-3)}}=-\frac{1}{8} \sqrt{\frac{91}{2 \pi }} \left(x^6-15 z^2 \left(x^4+y^4\right)+15 z^4 \left(x^2+y^2\right)+y^6-2 z^6\right) $$
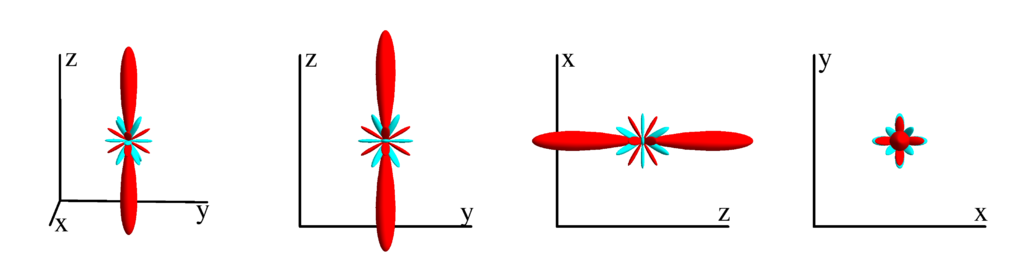
$m_l=-2$ - $t_{1g}$
$$ K_{6}^{(-2)}=\frac{3}{128} \sqrt{\frac{91}{\pi }} \sin (\theta ) \cos (\theta ) \left(-11 \sin ^4(\theta ) \sin (5 \phi )+5 \sin ^2(\theta ) \left(3-11 \cos ^2(\theta )\right) \sin (3 \phi )+2 \left(33 \cos ^4(\theta )-30 \cos ^2(\theta )+5\right) \sin (\phi )\right)\\ \phantom{K_{6}^{(-2)}}=-\frac{3}{8} \sqrt{\frac{91}{\pi }} y z (y-z) (y+z) \left(-10 x^2+y^2+z^2\right) $$
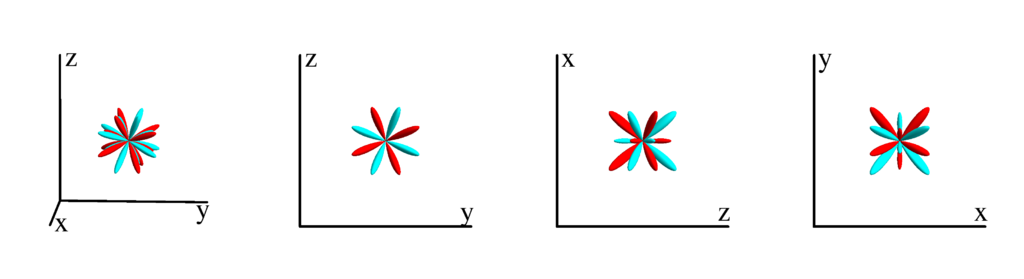
$m_l=-1$ - $t_{1g}$
$$ K_{6}^{(-1)}=\frac{3}{128} \sqrt{\frac{91}{\pi }} \sin (\theta ) \cos (\theta ) \left(-2 \left(33 \cos ^4(\theta )-30 \cos ^2(\theta )+5\right) \cos (\phi )+5 \sin ^2(\theta ) \left(3-11 \cos ^2(\theta )\right) \cos (3 \phi )+11 \sin ^4(\theta ) \cos (5 \phi )\right)\\ \phantom{K_{6}^{(-1)}}=\frac{3}{8} \sqrt{\frac{91}{\pi }} x z (x-z) (x+z) \left(x^2-10 y^2+z^2\right) $$
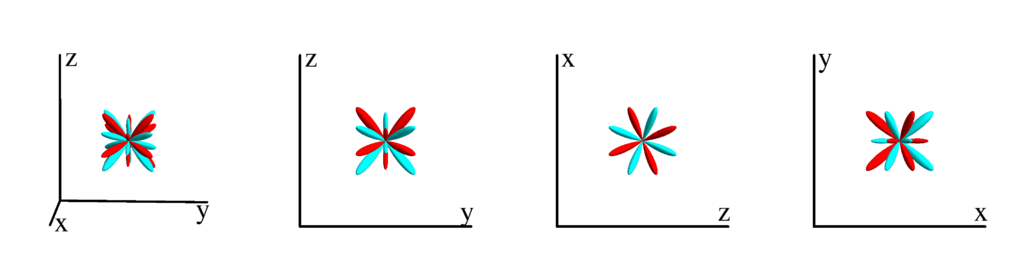
$m_l=0$ - $t_{1g}$
$$ K_{6}^{(0)}=\frac{3}{64} \sqrt{\frac{91}{\pi }} \sin ^4(\theta ) (11 \cos (2 \theta )+9) \sin (4 \phi )\\ \phantom{K_{6}^{(0)}}=-\frac{3}{8} \sqrt{\frac{91}{\pi }} x y (x-y) (x+y) \left(x^2+y^2-10 z^2\right) $$

$m_l=1$ - $t_{2g}^{(1)}$
$$ K_{6}^{(1)}=\frac{1}{256} \sqrt{\frac{1365}{2 \pi }} \sin (\theta ) \cos (\theta ) \left(33 \sin ^4(\theta ) \sin (5 \phi )+9 \sin ^2(\theta ) \left(3-11 \cos ^2(\theta )\right) \sin (3 \phi )+2 \left(33 \cos ^4(\theta )-30 \cos ^2(\theta )+5\right) \sin (\phi )\right)\\ \phantom{K_{6}^{(1)}}=\frac{1}{16} \sqrt{\frac{1365}{2 \pi }} y z \left(16 x^4-16 x^2 \left(y^2+z^2\right)+\left(y^2+z^2\right)^2\right) $$

$m_l=2$ - $t_{2g}^{(1)}$
$$ K_{6}^{(2)}=\frac{1}{256} \sqrt{\frac{1365}{2 \pi }} \sin (\theta ) \cos (\theta ) \left(2 \left(33 \cos ^4(\theta )-30 \cos ^2(\theta )+5\right) \cos (\phi )+9 \sin ^2(\theta ) \left(11 \cos ^2(\theta )-3\right) \cos (3 \phi )+33 \sin ^4(\theta ) \cos (5 \phi )\right)\\ \phantom{K_{6}^{(2)}}=\frac{1}{16} \sqrt{\frac{1365}{2 \pi }} x z \left(x^4+2 x^2 \left(z^2-8 y^2\right)+16 y^4-16 y^2 z^2+z^4\right) $$
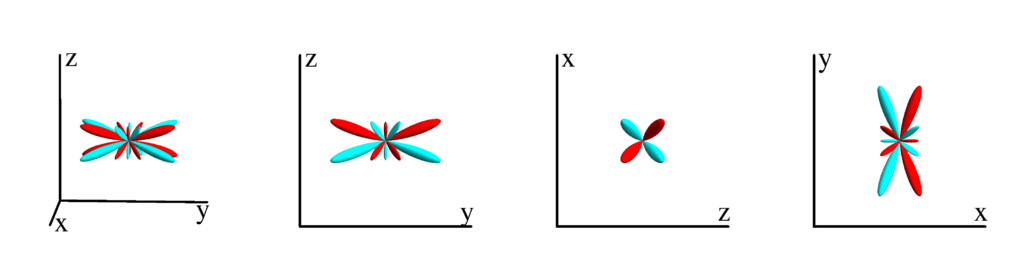
$m_l=3$ - $t_{2g}^{(1)}$
$$ K_{6}^{(3)}=\frac{1}{256} \sqrt{\frac{1365}{2 \pi }} \sin ^2(\theta ) (60 \cos (2 \theta )+33 \cos (4 \theta )+35) \sin (2 \phi )\\ \phantom{K_{6}^{(3)}}=\frac{1}{16} \sqrt{\frac{1365}{2 \pi }} x y \left(-16 z^2 \left(x^2+y^2\right)+\left(x^2+y^2\right)^2+16 z^4\right) $$
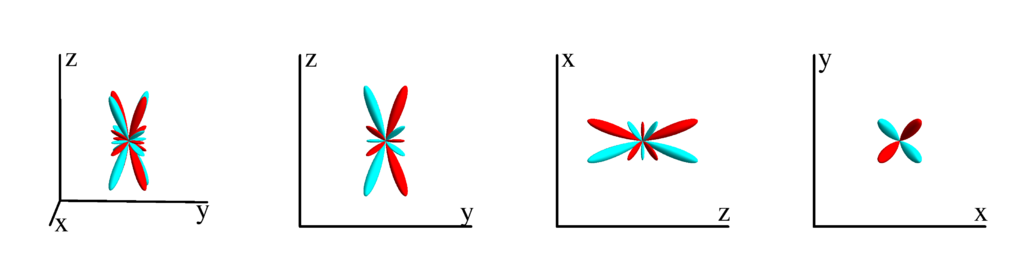
$m_l=4$ - $t_{2g}^{(2)}$
$$ K_{6}^{(4)}=\frac{\sqrt{\frac{3003}{2 \pi }} \left(3 (5 \sin (2 \theta )+12 \sin (4 \theta )+33 \sin (6 \theta )) \sin (\phi )+48 \sin ^5(\theta ) \cos (\theta ) \sin (5 \phi )+20 \sin ^3(\theta ) (21 \cos (\theta )+11 \cos (3 \theta )) \sin (3 \phi )\right)}{4096}\\ \phantom{K_{6}^{(4)}}=\frac{1}{16} \sqrt{\frac{3003}{2 \pi }} y z \left(3 y^4-10 y^2 z^2+3 z^4\right) $$

$m_l=5$ - $t_{2g}^{(2)}$
$$ K_{6}^{(5)}=\frac{\sqrt{\frac{3003}{2 \pi }} \left(240 \sin (2 \theta ) \sin ^4(\phi ) \cos (\phi )+48 \sin (4 \theta ) \sin ^2(\phi ) (7 \cos (\phi )+\cos (3 \phi ))+\sin (6 \theta ) (198 \cos (\phi )+55 \cos (3 \phi )+3 \cos (5 \phi ))\right)}{8192}\\ \phantom{K_{6}^{(5)}}=\frac{1}{16} \sqrt{\frac{3003}{2 \pi }} x z \left(3 x^4-10 x^2 z^2+3 z^4\right) $$
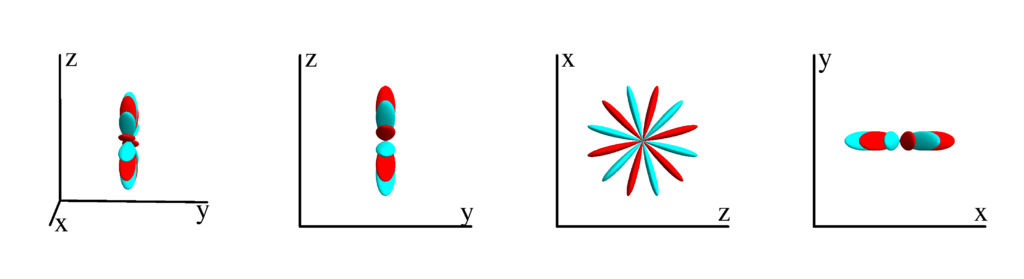
$m_l=6$ - $t_{2g}^{(2)}$
$$ K_{6}^{(6)}=\frac{1}{32} \sqrt{\frac{3003}{2 \pi }} \sin ^6(\theta ) \sin (6 \phi )\\ \phantom{K_{6}^{(6)}}=\frac{1}{16} \sqrt{\frac{3003}{2 \pi }} x y \left(3 x^4-10 x^2 y^2+3 y^4\right) $$